الگوی سوزوکی نظریه نقاط ثابت

 

 

چکیده

در این مقاله با استفاده از رویکرد الگوی سوزوکی، شرایط قراردادی جدید را در چارچوب فضاهای متریک فازی نا ارشمیدسی معرفی می کنیم.

ما همچنین قضیه نقطه ثابت تصادفی مربوط به دو نگاشت در این چارچوب را اثبات می کنیم. در پایان، دو مثال برای اثربخشی و کاربردی بودن نتایج اصلی ما ارائه شده است.

 

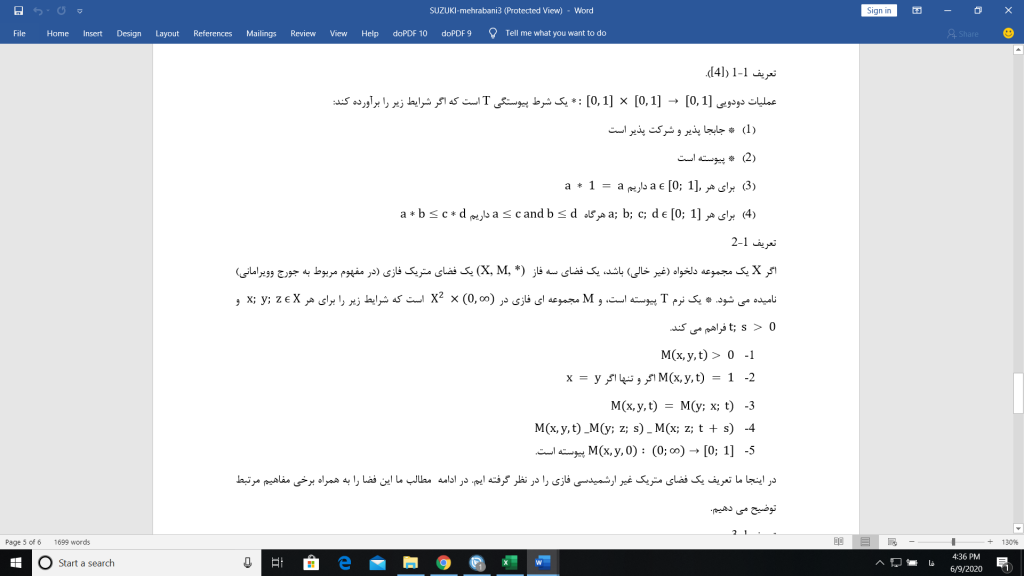
اصل انقباض باناخ [1] انگیزه بسیاری از مطالعات نقطه ثابت در فضاهای مختلف بود ([6] {[11] ، [16] ، [22]، [25]، [30]). در سال 2008 سوزوکی تعمیم جالبی برای اصل نقطه ثابت باناخ ارائه داد.

قضیه 1-1 ([26]). فرض کنید  یک فضای متریک کامل بوده و  نگاشتی روی باشد.

 

 

 

 

ABSTRACT

In this paper by using of Suzuki-type approach we introduce the new contractive condition in the framework of non-Archimedean fuzzy metric spaces.

We prove also the corresponding coincidence fixed point theorem for two mappings in this framework.

Finally, two examples are presented to verify the effectiveness and applicability of our main

results.

Banach contraction principle [1] was motivation for many fixed point studies in various spaces

In 2008 Suzuki introduced an interesting generalization of Banach fixed point principle.

 

الگوی سوزوکی نظریه نقاط ثابت

This theorem was later generalized in.

In addition to the above results, Kikkawa and Suzuki [13] provide a Kannan type version of the theorems mentioned before. In [21], it is provided a Chatterjea type version.

Popescu [20] gives a Ciric type version. Recently, Kikkawa and Suzuki also provide multivalued versions which can be found in

The aim of this paper is to present some Suzuki-type of common fixed point result for two mappings under generalized contractive condition in fuzzy metric space.

Before starting and proving our results, we present some definitions and propositions in fuzzy metric space.

 

برای مشاهده مطالب بیشتر به سایتwww.farzdon.ir مراجعه نماید. 

الگوی سوزوکی نظریه نقاط ثابت