درخت های شبه متریک
درخت های شبه متریک
نگارش مقاله ریاضی
چکیده:
بررسی درخت های متریک توسط ج.تیتس در سال 1977 آغاز گردید.
اخیرا ما مفهوم عمومی تری از درخت متریک را مطالعه کردیم.
در مقاله پیش رو، در بین سایر واقعیت ها ما اثبات کردیم که پوش ابر محدب q از یک درخت شبه متریک ابر محدب q خود یک درخت شبه متریک است.
این بدون استفاده از ویژگی چهار گوش به دست آمده است، یک مفهوم هندسی که توسط آکسوی و ماریزی به کار گرفته شده است تا نشان دهد هر درخت متریک کامل ابر محدب است.
مقدمه
درختان متریک از سال 1977 مورد مطالعه قرار گرفته است.
(نگاه کنید به [11] ج. تیتس، یک قضیه کالچین برای درختان ، مشارکت در جبر: مجموعه مقالات اختصاص داده شده به الیس کلچین ، آکادمیک پرس ، نیویورک ، 1977).
برخی نتایج در زمینه درختان متریک کاربردهایی در بسیاری زمینه های ریاضیات مانند هندسه ، توپولوژی و نظریه گروه پیدا کرده است.
اهمیت درختان متریک محدود به زمینه های ریاضی نیست، به طور مثال مطالعه درختان فیلوژنتیک در زیست شناسی و پزشکی همچنین نظریه درختان متریک را به کار می گیرد.
(به [10] سی.سمپل، ام. استیل، فیلوژنتیک، سری سخنرانی دانشگاه آکسفورد در ریاضیات و کاربردهای آن ، 24 2003 مراجعه کنید).
کاربردهای درختان متریک در علم اطلاعات، به ویژه در علوم رایانه [3 ، 6] انگیزه های بزرگی برای تعمیم نتایج درختان متریک از یک مجموعه متقارن گرفته تا یک چارچوب نامتقارن است.
پس این پروژه بخشی از آن مأموریت گسترده است.
در [4] ، درس اثبات کرد که هر درخت متریک میانه است. در اینجا ، ما می توانیم این نتیجه که فرضیه با رها کردن شرایط تقارن تابع فاصله، ضعیف می گردد را دنبال کنیم.
علاوه بر این ، آکسوی و ماریزی در [3] با استفاده از خاصیت چهارگوش یک درخت متریک، ارتباط میان یک درخت متریک و پوش ابر محدب آن را بررسی کردند.
در [9] ، ما شروع به تحقیق در مورد مفهوم درخت متریک در فضاهای شبه متریک ، که آن را درخت شبه متریک می نامیم ، کردیم.
در این مقاله ، ما مطالعه خود را در مورد این مفهوم با تعمیم برخی از نتایج شناخته شده در مورد درختان متریک از مجموعه متریک تا دیدگاه شبه متریک ادامه می دهیم.
به ویژه، ما اثبات می کنیم که پوش ابر محدب q از یک درخت شبه متریک یک درخت شبه متریک است.
در میان سایر نتایج ما نتیجه آگینی و همکارانش که می گوید وقتی فضای شبه متریک به یک پیوستگی می پیوندد، هر یک از نقاط انتهایی آن نقطه انتهایی از پوش ابر محدب q خودش است، را تعمیم می دهیم.
همچنین نمونه های مختلفی در این مقاله برای نشان دادن مفاهیم موجود در مطالعه، ارائه شده است.
بطور شگفت آوری، تحقیقات ما نشان می دهد که بسیاری از نتایج کلاسیک در مورد درختان متریک نیازی به استفاده از تقارن تایع فاصله ندارند.
از این رو اگرچه گهگاه به شکلی متفاوت اما آنها در یک موقعیت شبه متریک قرار می گیرند.
انجام پایان نامه ریاضی
پیش نیازها
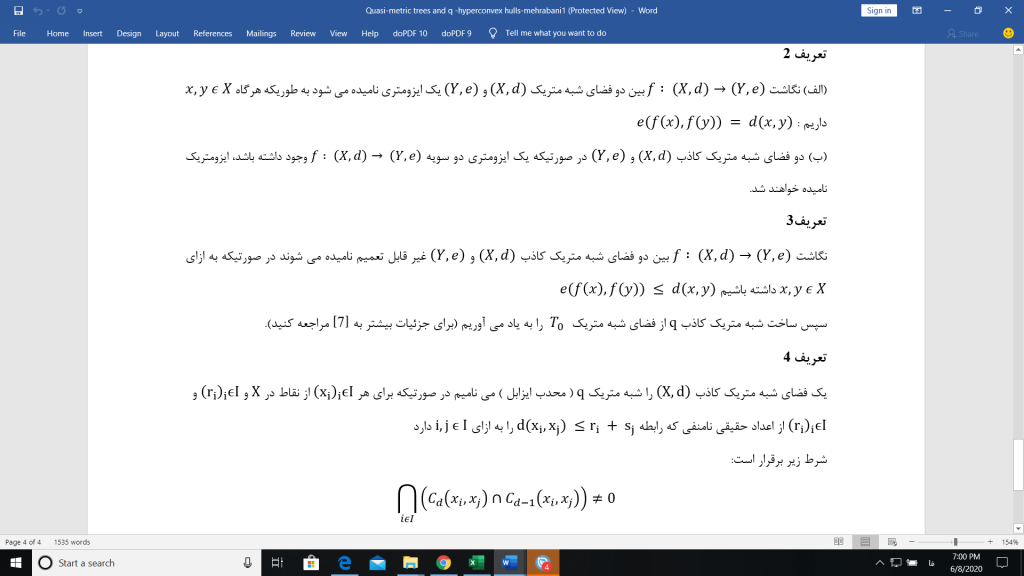
این بخش بعضی تعاریف مهم که در ادامه مقاله استفاده خواهیم کرد را یاداوری می کند.


درخت های شبه متریک
Abstract:
The investigation of metric trees began with J.
Tits in 1977. Recently we studied a more general notion of quasi-metric tree.
In the current article we prove, among other facts, that the q –hyper convex hull of a q –hyper convex T0 -quasi-metric tree is itself a T0 -quasi-metric tree.
This is achieved without using the four-point property, a geometric concept used by Aksoy and Maurizi to show that every complete metric tree is hyper convex.
Introduction
Metric trees have been studied since 1977 (see [11] J. Tits, A theorem of Lie-Kolchin for trees, Contributions to Algebra: a collection of papers dedicated to Ellis Kolchin, Academic Press, New York, 1977).
A number of results on metric trees have found applications in many fields of mathematics like geometry, topology, and group theory.
The importance of metric trees is not limited to mathematics, as for instance the study of phylogenetic trees in biology and medicine also employs metric trees (see [10] C. Semple, M. Steel, Phylogenetics, Oxford University Lecture Series in Mathematics and its Applications, 24 2003).
The applications of metric trees in information science, particularly in computer science [3, 6], are great motivations for the generalization of results about metric trees from a symmetric setting to an asymmetric
framework. Thus this project is a part of that broad mission.
In [4], Dress proved that any metric tree is median.
Here, we establish that this result still holds when the hypothesis is relaxed by dropping the symmetry condition of the distance function.
Moreover, Aksoy and Maurizi in [3] studied the relationship between a metric tree and its hyper convex hull by using the four-point property of a metric tree.
In [9], we started investigating the concept of a metric tree in T0 -quasi-metric spaces, which we called quasi-metric tree.
In this article, we continue our study of this concept by generalizing some well-known results about metric trees from metric setting to the quasi-metric point of view.
In particular, we prove that the q –hyper convex hull of a T0 -quasi-metric tree is a T0 -quasi-metric tree.
Among other results, we also extend the result of Agyingi et al., which says that when a T0 -quasi-metric space is join compact then each of its endpoints is an endpoint of its q –hyper convex hull.
Several examples are also provided in this paper to illustrate the concepts involved in the study.
Surprisingly, our investigations reveal that many classical results about metric trees do not require the use
of symmetry of distance function. Hence they hold in a quasi-metric setting, although sometimes in a slightly different form.
انجام مقاله ریاضی
برای مشاهده مطالب بیشتر به سایت www.farzdon.ir مراجعه نماید.